Physics 221

ELEMENTS OF PHYSICS I
Physics 221

SAMPLE TEST 2 SOLUTIONS

Problem 1 (10 points)

(a)

Explain why you tend to lean backward when carrying a heavy load in your arms. Draw a picture showing the forces to support your explanation.

ANSWER: The heavy load applies a torque on you (clockwise). You need to lean backward to counterbalance that torque with the torque of your weight (counterclockwise).

(b)

Can a centripetal force ever do work on an object? Explain.

ANSWER: The centripetal force is always perpendicular to the direction of motion. Only the component of the force in the direction of motion can do work. The centripetal force has no such component, so it can never do work.

(c)

A record turntable starts to rotate with increasing angular velocity. Does a point on the rim have radial and/or tangential acceleration? If the angular velocity increases uniformly, which component of the acceleration changes and why?

ANSWER: It has both radial (centripetal) acceleration

$\begin{displaymath}a_R = \frac{v^2}{r} = \omega^2 r\end{displaymath}$

and tangential acceleration

$\begin{displaymath}a_t = r\alpha\end{displaymath}$

where $\alpha$ is the angular acceleration.

If the angular velocity increases uniformly, then $\alpha$ is constant, so the tangential acceleration does not change. The radial acceleration increases, because the angular velocity $\omega$ increases.

(d)

When you release an inflated but untied balloon, why does it fly across the room?

ANSWER: Air escapes from the balloon that carries momentum. By momentum conservation, the balloon acquires momentum which is exactly opposite to the momentum of the escaping air. Therefore, it flies in a direction which is opposite to the velocity of the escaping air.

(e)

Explain what keeps a satellite up in its orbit around the earth.

ANSWER: The weight of the satellite is being used as a centripetal force. In fact, the satellite is in a state of free fall because of its weight, but never reaches the ground because all the weight can do is change the direction of the tangential velocity.

$\begin{displaymath}W = m\frac{v^2}{r}\end{displaymath}$

Problem 2 (10 points)

A 20-g bullet traveling 300 m/s hits a 1.5-kg block of wood which is stationary on a frictionless surface and penetrates it. After the collision, the block moves with speed 0.8 m/s.

(a)

What is the speed of the bullet after the collision?

The momentum before the collision is

$\begin{displaymath}p = mv + MV = (0.02~kg)\times (300~m/s) + (1.5~kg)\times (0~m/s) = 6~kg.m/s\end{displaymath}$

The momentum after the collision is

$\begin{displaymath}p = mv' + MV' = (0.02~kg)v' + (1.5~kg)\times (0.8~m/s) = (0.02v' +1.2)~kg.m/s\end{displaymath}$

Momentum is conserved, so

p=p'

Solving for v', we obtain

$\begin{displaymath}v' = \frac{6-1.2}{0.02} = 240~m/s\end{displaymath}$

(b)

What was the impulse on the bullet?

The impulse is

$\begin{displaymath}I = F\Delta t = \Delta p = mv' - mv = m(v'-v) = 0.02\times (240-300) = -1.2~N.s\end{displaymath}$

(c)

How much energy was lost in the collision?

The kinetic energy before the collision is

$\begin{displaymath}\mathrm{KE} = {\textstyle{\frac 1 2}} mv^2 + {\textstyle{\fra... ...^2 = {\textstyle{\frac 1 2}}\times 0.02\times 300^2 + 0 = 900~J\end{displaymath}$

The kinetic energy after the collision is

$\begin{displaymath}\mathrm{KE}' = {\textstyle{\frac 1 2}} m{v'}^2 + {\textstyle{... ... 240^2 + {\textstyle{\frac 1 2}} \times 1.5\times 0.8^2 = 576~J\end{displaymath}$

The energy lost is

$\begin{displaymath}-\Delta (\mathrm{KE}) = \mathrm{KE} - \mathrm{KE}' = 900 - 576 = 324~J\end{displaymath}$

Problem 3 (10 points)

(a)

It is an icy winter night and you just drove back home. To park your 1200-kg car, you need to go up your driveway, which has an incline of 15^o and is 10 m long. If you ignore friction and your weight, how much work is done as you go up your driveway?

You do work because the potential energy changes, so

W = mgh

where h is the height,

$\begin{displaymath}h = (10~m)~\sin 15^o = 2.59~m\end{displaymath}$

Therefore,

$\begin{displaymath}W = 1200\times 9.81\times 2.59 = 30490~J\end{displaymath}$

(b)

On a hot summer day, your driveway has a coefficient of friction $\mu = 0.2$ . How much work is done as you go up your driveway in this case?

In addition to the potential energy, you do work because of the friction, which is

$\begin{displaymath}F = \mu N\end{displaymath}$

where N is the normal,

$\begin{displaymath}N = mg\cos 15^o = 1200\times 9.81~\cos 15^o = 11371~N\end{displaymath}$

The friction is

$\begin{displaymath}F = 0.2\times 11371 = 2274~N\end{displaymath}$

The additional work is

$\begin{displaymath}W_F = 2274\times 10 = 22740~J\end{displaymath}$

The total work is

W_total = 30490 + 22740 = 53230 J

Problem 4 (10 points)

A 50-kg box is hanging from the ceiling as shown. Find the tension in the two chords supporting the box.

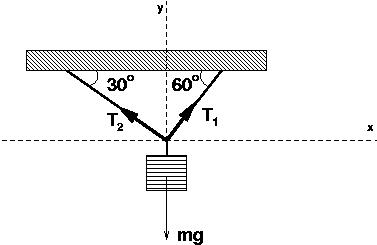
$\includegraphics{fig1s.pdf}$

x-components:

$\begin{displaymath}\sum F_x = T_1\cos 60^o - T_2\cos 30^o = 0\end{displaymath}$

y-components:

$\begin{displaymath}\sum F_y = T_1\sin 60^o + T_2 \sin 30^o - mg = 0\end{displaymath}$

Solve first equation for T₂,

$\begin{displaymath}T_2 = T_1\; \frac{\cos 60^o}{\cos 30^o}\end{displaymath}$

and plug in second equation

$\begin{displaymath}T_1\; \left( \sin 60^o + \frac{\cos 60^o \sin 30^o}{\cos 30^o}\right) -mg =0\end{displaymath}$

Solve for T₁,

$\begin{displaymath}T_1 = \frac{mg}{\sin 60^o + \frac{\cos 60^o \sin 30^o}{\cos 30^o}} = \frac{50\times 9.81}{1.155} = 424.7~N\end{displaymath}$

and for T₂,

$\begin{displaymath}T_2 = 424.7\; \frac{\cos 60^o}{\cos 30^o} = 245.2~N\end{displaymath}$

___________________________________________________

Back to home page Physics Department

401 Nielsen Physics Bldg., The University of Tennessee, Knoxville, TN 37996-1200, U.S.A.